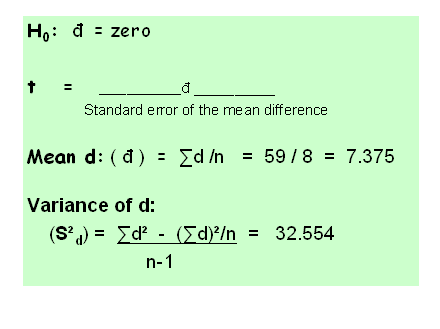
T-test for PAIRED DATA

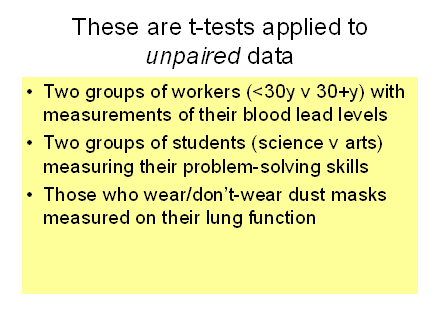
BUT in many cases the data are "paired" between the two groups:

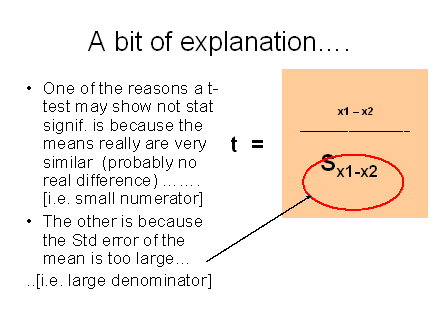
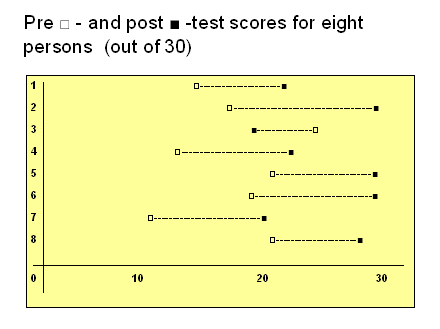
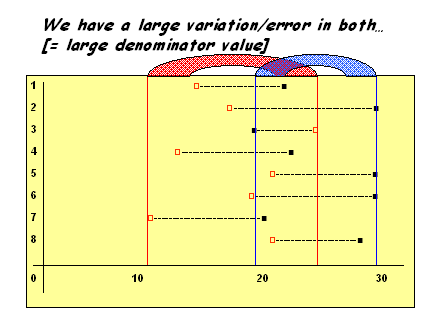
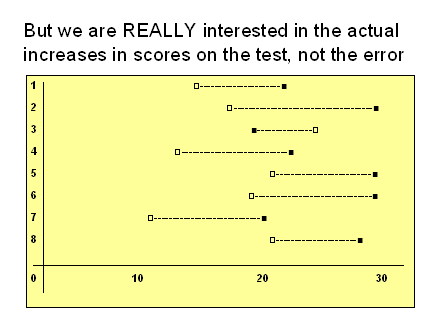
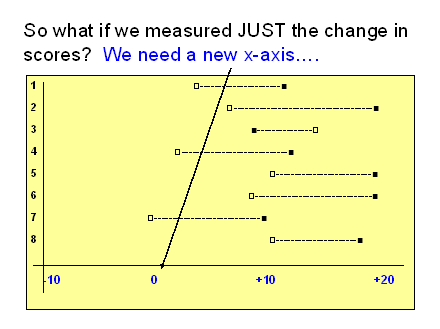
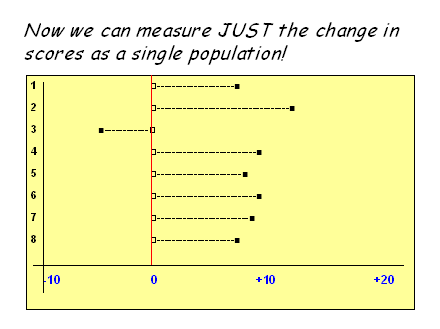
With MUCH less random error! (The denominator of the t-value)

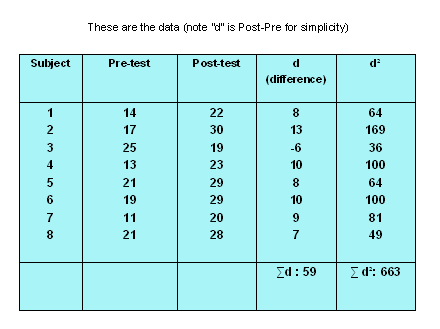
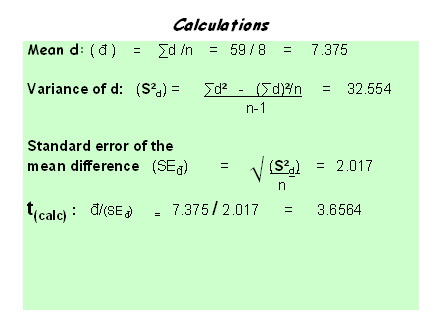
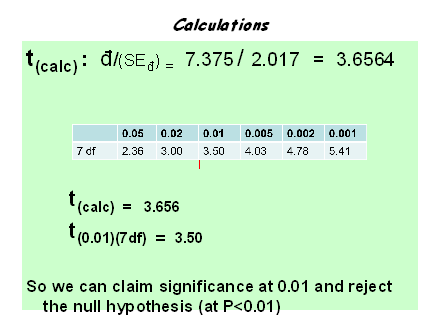
ON THE FACE OF THE INFORMATION GIVEN, IT SEEMS APPROPRIATE TO ANALYZE WITH A T-TEST FOR UNPAIRED DATA.  BUT IF IT IS LEARNED THAT THE DATA ARE FROM *FIVE* PEOPLE, IN A PRE- AND POST DESIGN, THEN A PAIRED ANALYSIS WOULD BE APPROPRIATE.  YOU DO NOT HAVE THE CHOICE.  "PAIRED" DATA REQUIRED PAIR T-TEST.  OTHERWISE THE UNPAIRED T-TEST MUST BE USED.   HOWEVER, HERE ARE BOTH METHODS APPLIED TO THE SAME SET OF DATA FOR ANALYSIS.  

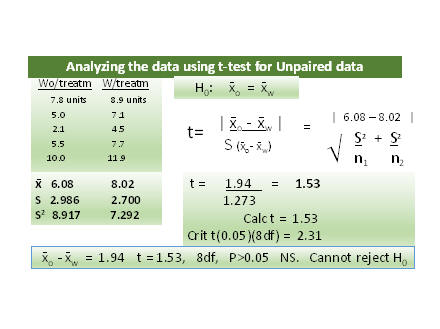
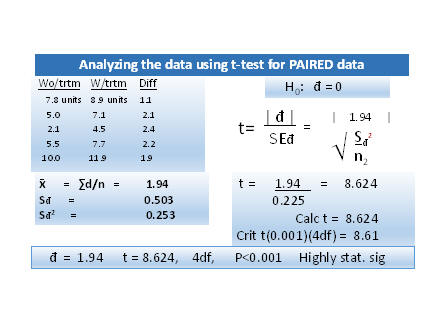
Here you can see the effect of the paired analysis.   The actual differences between pre- and post (whichever way you calculated it) was the same: 1.94.  The denominator was very large in the UNpaired test but very small in the paired test, resulting in the different t values.  The large SE of the mean in the UNpaired was due to the large amount of variation between the members of each group, but in the paired test, that variation was eliminated.